Punters seem obsessed with trifectas these days and I guess it's no surprise. Don Scott has seen the value in them and now everyone else is chasing it.
But, despite all that has been written, many punters still lack the basic knowledge that's needed to come to grips 'technically' with the trifecta method itself. Few punters progress beyond the three-horse $6 box.
The idea is this article is to refresh your memory about basic trifecta boxes and to throw in an idea or two about using 'banker' horses, or greyhounds, when you bet a trifecta. It'll be worth your while studying this article and filing it for future reference because there is no doubt the trifecta DOES offer the opportunity of a big return, if only you put enough time into your thinking.
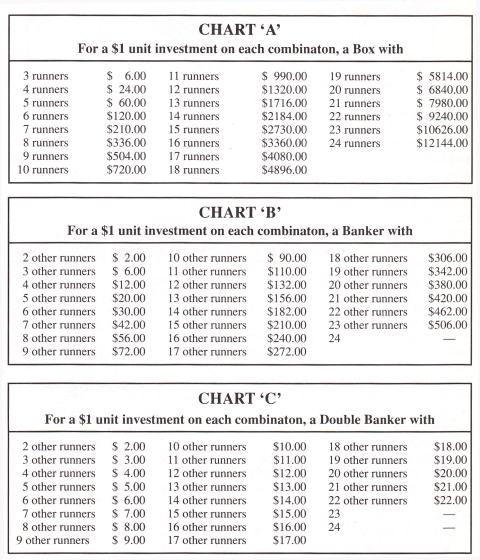
For a start, there's the normal box pattern. You select three or more horses and couple them in all possible combinations. This is fine, except that it quickly becomes far too expensive to link too many horses together, as Chart 'X reveals.
Few smaller-bet punters go beyond boxing four horses, even if they can bet only in 50 cent units. Big punters are braver, of course. They'll link eight or nine together if they reason that the potential payout is thousands of dollars.
Don Scott links up to five or six but he places different amounts of money on each combination, according to the chance he thinks it has of success.
Now we come to the 'banker' idea and the first idea is where you select a runner to win the race. You then select two or more runners to finish 2nd and 3rd. This is a fine idea, as long as you are confident of your banker bet.
I know a chap who took At Talaq as a banker in last year's Melbourne Cup and linked 10 other runners with it to finish 2nd and 3rd. It cost him $90 but he got a return of $14,000+. So it can really pay off, if you've got the courage of your convictions.
You can also use the 'banker' in another way: Pick it to run 2nd and then take your other multiple horses to win and run 3rd. Alternatively, you could use your banker to finish 3rd and take other horses to fill Ist and 2nd. Chart 'B' shows you what 'banker' trifecta bets cost:
Now we come on to the double banker trifeetas. A double banker is where you select one runner to win the race and another runner to finish 2nd. You then select two or more runners to finish 3rd. This is a top idea only when you are supremely confident of selecting 1st and 2nd with only two picks. Its advantage is that it is a cheap way of taking trifectas, as Chart 'C' shows.
Perhaps one of the most interesting but little-used trifecta methods is the multiple trifecta. What is a multiple? You pick as many runners as you like to come first, as many as you like to finish 2nd and as many as you like to finish 3rd. The costs of these types of bets vary according to the number of runners you have chosen for each placing.
There is a way of determining how much a bet will cost. It may seem a little difficult at first, but I can assure you that after a go or two you won't have any problems. Let's take an example of you taking runners 4 and 5 to win, 4, 5 and 6 to run 2nd and 4, 5, 6 and 7 to run 3rd. This is how you determine how much such a bet will cost:
MULTIPLY:
Number of selections for first place (2) x number of selections for second place (3) x number of selections for third place (4) which equals 24. Now you look for those horses which are in ALL three legs of the trifecta- in this case, numbers 4 and 5 and you multiply those two horses by two which equals 4. This is added to the 24 you already have to make 28.
Your next step is as follows:
MINUS:
The number of selections for first place (2) is multiplied by the number of selections common to 2nd and 3rd; in this instance three of them. So, you have 2 x 3 = 6. You now look at the number of selections for second place (3) and this figure is multiplied by the number of selections common to 1st and 3rd places, which in this instance is two. So we have 3 x 2 = 6 again. The final thing is to look at the number of selections for third place, which is in this case 4 and multiply that figure by the number of selections common to 1st and 2nd places, in this case 2. So we have 4 x 2 = 8.
We add these three figures together (6 + 6 + 8) and this equals 20. You now deduct 20 from your original figure of 28 and this leaves you 8, which tells you that the multiple bet we have looked at will $8 (assuming $1 units).
If there are no common selections (that is, you have completely different horses in each kg of the trifecta) all trifectas would be singles and their cost is calculated simply by multiplying the number of selections for first, second and third.
I believe the multiple trifecta idea has a lot going for it and I urge you to examine the possibility of using it in conjunction with your normal 'box' trifecta betting.
By Jon Hudson
PRACTICAL PUNTING - MARCH 1987